Teachers often get asked “What makes you different?”. You’d probably get a different answer from each of my students but there is something in particular that runs through all my lessons: I believe in teaching from the basics, building up knowledge, and sorting out problems as they arise. Trigonometry is often perceived as a scary topic in mathematics, but I don’t believe it should be.
If you try an internet search for “I hate trigonometry”, it will bring up a scary number of results! There’s even a music video entitled I hate trigonometry! This is a very sad situation because trigonometry does not need to be so scary. I don’t believe that any section of mathematics should have this label; all maths should be structured and taught progressively so students are doing “hard things” before they realise it.
A quick internet search about teaching trigonometry sent me heading for the kettle in desperate need of a cup of tea! There is a wealth of opinions out there: trigonometry should be removed from the syllabus, trigonometry is a silly section of math, trigonometry is taught badly, and trigonometry made me hate math. But there’s also a wealth of ideas for trying to teach trigonometry differently.
I teach trigonometry as part of the CambriLearn AS Level Pure Maths P1 course. This article will take you through an overview of the section to illustrate how I start with a gradual introduction and work up to the complex work. Students are happy and working strongly, and then they realise that they’re doing “difficult” things!
Trigonometry section overview
I haven’t yet found a textbook that teaches trigonometry in the way that I “want it to”. I believe in teaching technique, but I believe in teaching technique with understanding. The technique must link to things the student already knows so that they can scaffold the new knowledge and understand how it connects back.
The AS Level Pure Maths P1 syllabus covers the following skills:
- sketch and use graphs of the sine, cosine and tangent functions (for angles of any size, and using either degrees or radians)
- use the exact values of the sine, cosine and tangent of 30 degrees, 45 degrees, 60 degrees and related angles
- use the notations sin-1x, cos-1x, tan-1x to denote the principal values of the inverse trigonometric relations
- use the identities sinx/cosx= tanx and sin2x+ cos2x= 1
- find all the solutions of simple trigonometrical equations lying in a specified interval (general forms of solution are not included)
This may seem basic when compared with the lists of topics of other courses, and even against the full A Level Maths syllabus. However, this is a great grounding in trigonometry understanding. If I can get this nailed down with my students, their future trigonometrical life becomes so much easier.
I cover trigonometry in 11 lessons over 21 academic days. I don’t believe that students should be learning a new section every day they do school work, especially for AS and A Level Math, because a lot of practice is required. Students need time for the knowledge and understanding to marinade in their brain and settle in. It takes time to practise and really get to grips with the information.
Setting up the basics
Before starting with AS Level trigonometry, I spend a day doing revision of IGCSE Math trigonometry. I like to make sure that the trigonometry ratios (SOHCAHTOA!), and Pythagorean theorem are all solid in my students’ minds.
The first step into AS trigonometry comes with special angles, as these are directly and easily linked to IGCSE Math work. Working within familiar triangles helps students to calm their trigonometry fears… AS trigonometry is not so bad after all!
After special angles comes trig identities. This starts the brain stretching process and brings the understanding that there is a lot more to trigonometry than SOHCAHTOA. This is also a great time to practise fraction algebra and factorisation. It’s always brilliant to consolidate earlier skills!
If I were teaching trig identities in a classroom, I would be constantly talking through what I am doing at each step. To translate this into an online course format, I make sure that each and every step of my examples is clearly annotated. Colour is also an important ingredient for teaching maths online!

Getting to know trig graphs
After lots and lots of trig identities, comes the time to expand on the idea that there’s more to trig than 90 degrees. The trig graphs are the best way to get this across, and I believe that understanding trigonometrical equations is so much easier if you understand the graphs. I therefore spend the first two lessons of my equations section just in getting to know the graphs. A whole lesson is spent on the shapes of the graphs and the concept of periodicity.
Once the graphs are concrete, the move is made into transformations of the trigonometry graphs. Here, I link straight back to working with quadratics. Linking back to early topics helps with reinforcement of prior knowledge and helps to reassure students that they are still in familiar maths territory. I am very thorough with quadratics at the beginning of the course because they factor into so many topics later on.
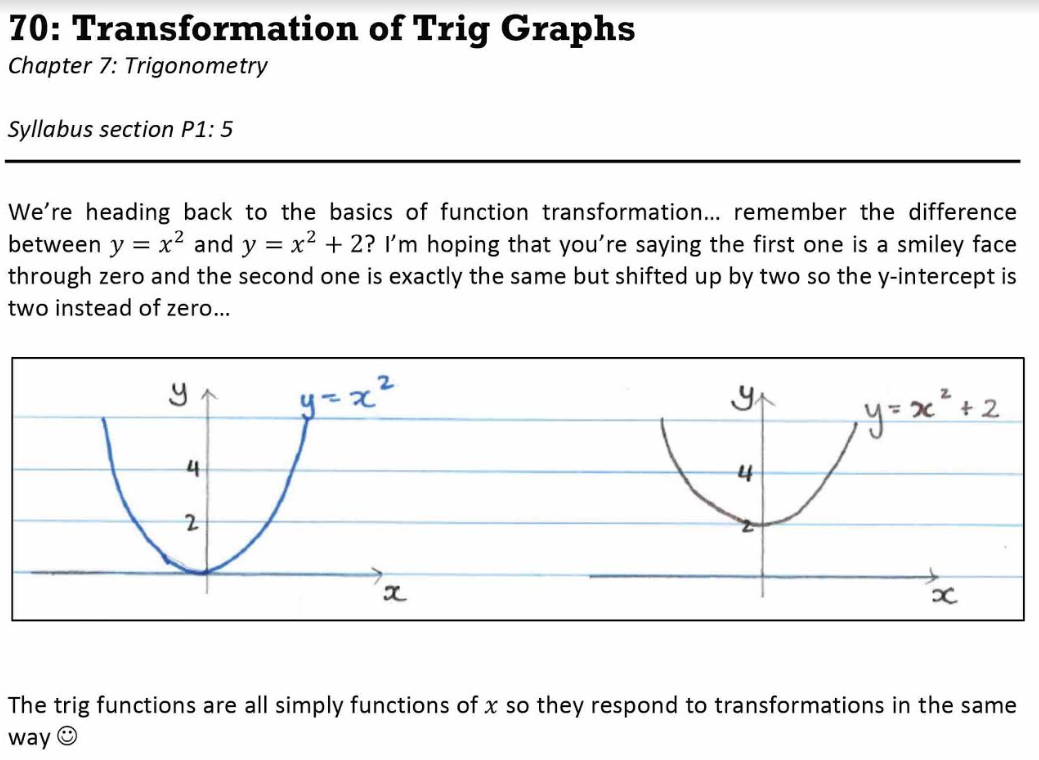
After revision of transformation of quadratics, and some revision of the basics of functions, we move into transformation of trigonometry graphs. In order to get students fully comfortable with the transformations, there is a long list of examples, all annotated as usual.
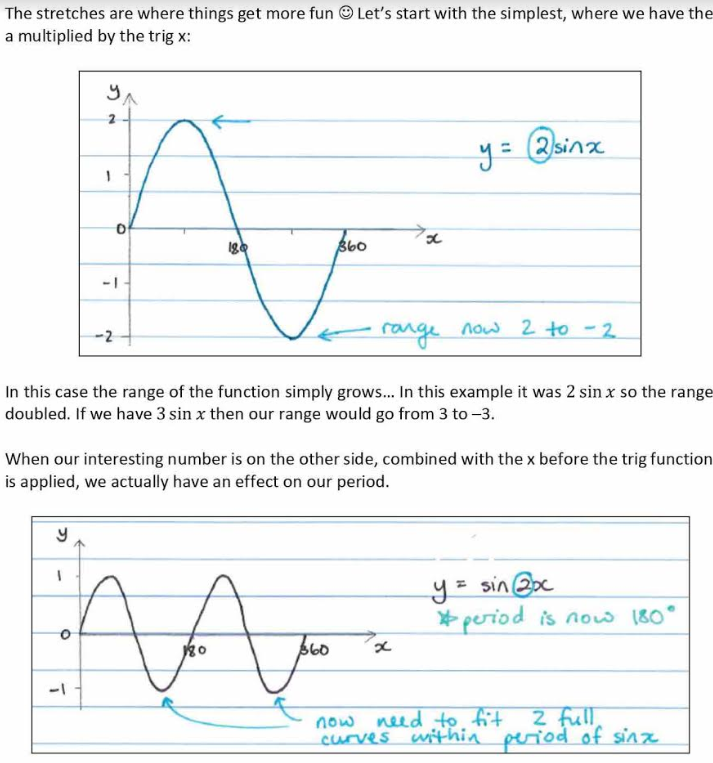
I also make full use of the CambriLearn Tech Teams’ skills in animation in this topic, as it is wonderful to be able to show students visually how the functions change shape for more complex transformations.
Building into trig equations
Students who are happy with the transformation of the trigonometry graphs are then ready to move towards solving trigonometry equations. The first step is revision of inverse functions… and one-to-one functions… before looking at inverse trigonometry. Usually by this time, students are more than ready to get to grips with an equation.
When I move into trigonometry equations, I split them into three different types:
- Simple trig
- Quadratic trig
- Transformed trig
Each type of equation is dealt with in a separate lesson, with questions specially selected from the textbook exercises that are relevant to that type. In order to help students organise their time and make sure that they are doing the correct questions, each week of my course has a breakdown of the work to be covered, including exercises and a minimum set of questions that must be done. I provide supplementary questions in areas where I think the students need more practice than is given in the textbook.
Each type of trigonometry equation has a specific approach to the solution, with variations of the approach for simple trigonometry equations. This enables the students to get to grips with the equations and get past any fear they may have with the increasing complexity. Each equation type is linked solidly to work that the students have already covered and become familiar with, so that they can progress more smoothly.
To start with, solving the simple trig equations is simplified into a series of steps. This gives the students a structure that helps them to remember all the steps involved in solving the equation.
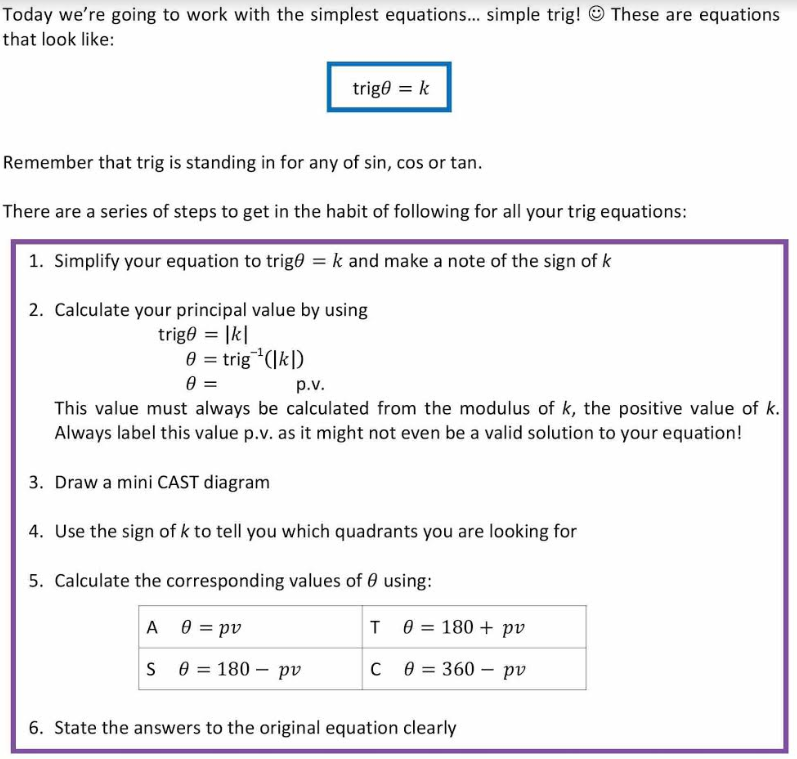
Whenever I give a series of steps, I always do an annotated example showing exactly how these steps appear in a question. It is important that the students have something solid to ‘grip onto’ rather than just a list of steps to follow.
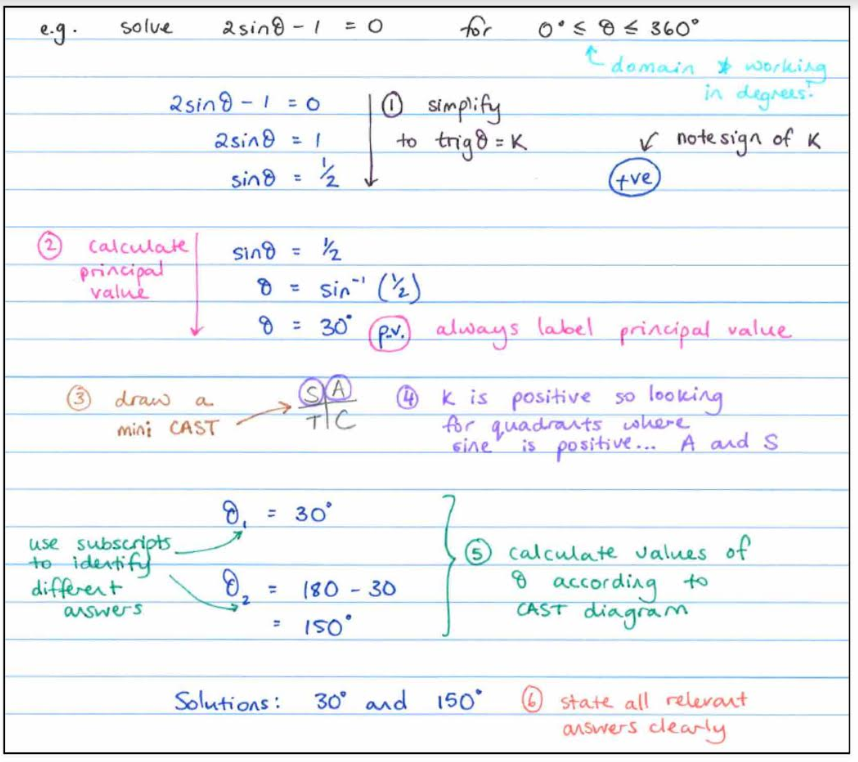
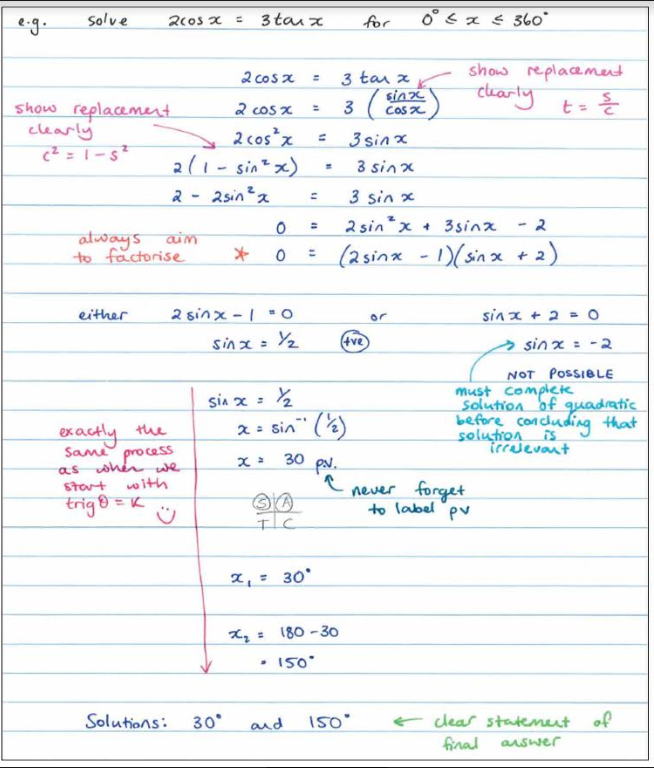
When I build up to the more complex trig equations, I simply connect other topics with this simple trig equation that the students have practised many times.
After working through all three types of equations in degrees, the students move straight into doing the equations in radians. Students have already been taught radians when they learned Circle Geometry in the previous section of the course. They are therefore ready to just switch over their calculators and do trigonometry in radians!
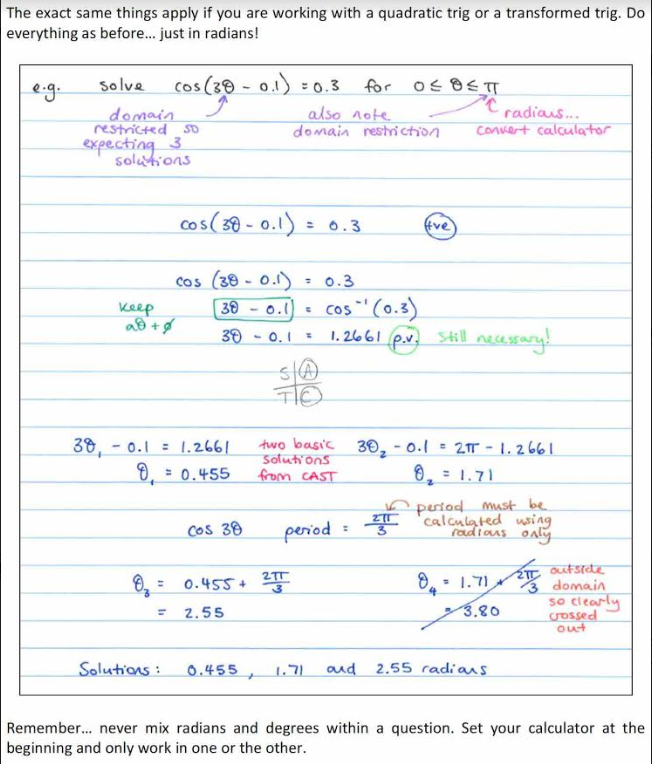
Again, having lots of practice in radians as well as in degrees, means that the students have ample opportunities to get comfortable with solving all the different types of equations that form part of their syllabus.
Changed your opinion of trig yet?
Perhaps after reading this, you’re simply reliving nightmares from high school… Perhaps after reading this, you’ve got a different idea of how trigonometry can be broken down… Hopefully, after reading this, you’ll understand that trigonometry does not have to be a scary experience!
If you want the full lessons (along with all the other AS Math lessons), you’re going to have to head over to CambriLearn and sign up…










